Dyson Spheres -- Background Information
Universe Background -- Dyson Spheres Information -- The Orellian System
Orell: Space City --
The 'Skyhook'
Aqua: Argo --
Vulka: Smith's Landing --
Technos --
Charan: Cicely --
Harvax: Chaross --
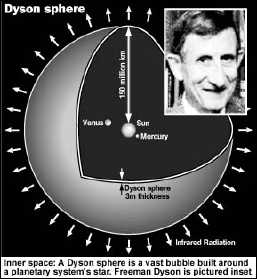 The
Ultimate Biospheres
The
Ultimate Biospheres
In 1937 Olaf Stapledon described
the concept of artificial biospheres constructed by advanced extraterrestrial
civilizations in his science fiction novel "Star Maker." About 20 years
later physicist Freeman Dyson formulated the idea that very advanced ET
civilizations may construct large artificial shells around their parent
star. These so-called "Dyson Spheres" would be capable of capturing all
of the star's energy for use by these civilizations. Advanced civilizations
are divided into three major classes by the exo-biology community. Type
I civilizations have the capability to communicate using electromagnetic
radiation and have a basic understanding of the laws of physics. They have
an energy capability equal to the solar insulation on Earth (between 1016
and 1017 watts). A Type II community has the capability of constructing
Dyson Spheres and can initiate interstellar travel and space colonization.
Their societal lifetimes are long, ranging from 1000 to 100,000 years.
The Galactic civilizations or Type III societies have energy resources
on the order of their entire galaxy (about 1044 ergs/sec). They have very
long lifetimes on the order of the main sequence lifetime of their sun.
They are effectively the "immortals" among the galactic communities. The
"Dyson Sphere" constructing civilizations would rank at the late evolutionary
stages of Type II societies in the transition phase to Type III communities.
The giant biosphere would
most likely be constructed from dismantled planets within the solar system
of the advanced civilization. According to Dyson, a large shell could be
constructed around the central star using the mass of a planet like Jupiter.
If the radius of the Dyson sphere is taken to be 1 astronomical unit (1
AU = the mean distance between the earth and the sun) it's volume would
be 4*pi*R^2*S, where R is the radius of the sphere (1 AU) and S thickness.
A shell or layer of rigidly built objects with a diameter of 10^6 moving
in orbits about the parent sun would require approximately 100,000 objects
to complete the spherical enclosure. Radiating at a temperature of 300
Kelvin this Dyson sphere would be a powerful source of infrared radiation.
Using Wein's law,
given by Lambda=0.29T-1,
the emitted radiation peaks at approximately 9.7 microns (in the infrared
region of the EM spectrum). Attempts to detect Dyson Spheres using the
IRAS (Infrared Astronomical Satellite) sky survey data are currently underway.
Information from: Mark Elowitz
The Dyson Sphere FAQ
by Anders Sandberg with ideas and additions from: Richard Treitel <treitel@wco.com> Stefan E. Jones <stefanj@io.com> Dani Eder < ederd@bcstec.ca.boeing.com> Cheradenine Zakalwe <zakalwe@vision25.demon.co.uk> Steve Linton <sal@caolila.dcs.st-and.ac.uk> David Lorenzo Duffy <dlduffy@welchlink.welch.jhu.edu> Erik Max Francis <max@alcyone.com> Frank Palmer <flpalmer@ripco.com> 'ric <btgsch@rmplc.co.uk> Christopher P. Winter <cpwinter@ix.netcom.com> Steve Willner <willner@cfa183.harvard.edu>
1. What is a Dyson Sphere?
The Dyson sphere (or Dyson shell) was originally
proposed in 1959 by the astronomer Freeman Dyson in "Search for Artificial
Stellar Sources of Infrared Radiation" in Science as a way for an advanced
civilisation to utilise all of the energy radiated by their sun. It is
an artificial sphere the size of an planetary orbit. The sphere would consist
of a shell of solar collectors or habitats around the star, so that all
(or at least a significant amount) energy will hit a receiving surface
where it can be used. This would create a huge living space and gather
enormous amounts of energy.
A Dyson sphere in the solar system, with a radius
of one AU would have a surface area of at least 2.72e17 km^2, around 600
million times the surface area of the Earth. The sun has a energy output
of around 4e26 W, of which most would be available to do useful work.
The original proposal simply assumed there would
be enough solar collectors around the star to absorb the starlight, not
that they would form a continuous shell. Rather, the shell would consist
of independently orbiting structures, around a million kilometres thick
and containing more than 1e5 objects. But various science fiction authors
seem to have misinterpreted the concept to mean a solid shell enclosing
the star, usually having an inhabitable surface on the inside, and this
idea was so compelling that it has been the main use of the term in science
fiction. The earliest appearance of this version seems to be Robert Silverberg's
novel Across a Billion Years.
A third kind of shell would be very thin and
non-rotating, held up by the radiation pressure of the sun. It would consist
of statites (see below, in the section about stability). Essentially it
is a "dyson bubble", where reflecting sails reflect light onto collectors
for use in external habitats. Its mass would be very smalll, on the order
of a small moon or large asteroid.
In the following I will call solid Dyson spheres Type II or dyson shells and independently orbiting spheres Type I.
2. Who is Freeman Dyson?
Freeman Dyson was born in 1923 in Crowthorne, Berkshire, England. Dyson received his bachelor of arts degree in mathematics from the University of Cambridge in 1945. He completed fellowships at Cambridge's Trinity College from 1946 to 1947, at Cornell University in 1947 and at the University of Birmingham from 1949 to 1951. He returned to Cornell to become a professor of physics in 1951, leaving in 1953 to join the Institute for Advanced Study, where he is now professor emeritus.
Dyson is a fellow of the Royal Society, a member of the U.S. National Academy of Sciences, a corresponding member of the Bavarian Academy of Sciences, a honorary fellow of Trinity College and an Associé Etranger de l'Académie des Sciences. He is president of the the SSI (Space Studies Institute).
Among his numerous awards and honors, Dyson received the Oersted Medal from the American Association of Physics Teachers, the Phi Beta Kappa Award in Science for Infinite in All Directions, the National Books Critics Circle Award for nonfiction, the 1981 Wolf Prize in physics, the Lewis Thomas Prize and many other honors.
Books by Dyson:
o Disturbing the Universe (1979)
o Weapons and Hope (1984)
o Infinite in all Directions (1988)
o Origins of Life (1986)
o From Eros to Gaia (1992)
o Selected Papers of Freeman Dyson (1996)
o Imagined Worlds (1997) Major papers on the net: Time Without End: Physics and Biology in an Open Universe
3. Was Dyson First?
No, he admitted himself that his original inspiration came from The Star Maker by Olaf Stapledon, written in 1937.
4. Why build a Dyson sphere?
Energy and space. As described above, the amount of collected energy would be immense, and the living space simply unimaginable. Dyson pointed out that so far the energy usage of mankind has increased exponentially for at least a couple of thousand years, and if this continues we will soon consume more energy than the Earth receives from the sun, so the natural step is to build artificial habitats around the sun so that all energy can be used. The same goes for population in the long run (it should be noted that this is not a solution, just a logical result of growth). It is also possible that the Dyson sphere simply stores the energy for future use, for example in the form of antimatter.
Even if cheap and efficient fusion power can be developed, eventually the waste heat has to be radiated away by a Dyson sphere-like cooling system.
Other proposed uses have been for security (although it is hard to hide the infrared emissions; energy could be radiated away in certain directions, but thermodynamics places some limits on it), or just for the fun of it (if you have a sufficiently advanced technology megaengineering could become a hobby activity; after all, ordinary people today perform engineering or crafting feats far beyond the imagination of previous eras).
5. What would a Dyson Sphere look like from the outside?
A Type I Dyson sphere would probably not cover the star perfectly, so occasional glimpses of its surface would be seen as the habitats orbited. A type II Dyson sphere would be totally opaque (unless it had openings). The spheres would hence be invisible from a distance, just a black disk on the sky. But they would shine powerfully in the infrared, as the waste heat from the internal processes radiate away. The apparent temperature would be T = (E / (4 pi r^2 eta sigma))^1/4
where E is the energy output of the sun, r the radius of the sphere, eta the emissivity and sigma the constant of Stefan-Boltzman's law.
This would correspond to an infrared wavelength of lambda = 2.8978e-3 / T m (assuming a blackbody sphere) which for reasonable sizes lies in the infrared. Dyson predicted the peak of the radiation at ten micrometers.
6. What would a Dyson Sphere look like from the inside?
The curvature of the "ground" would be even less than on Earth, so to an observer close to it it would look perfectly flat. In a solid dyson sphere with atmosphere, the atmosphere would limit the range of sight due to its opacity, and the horizon would be slightly misty.
The sky would be filled with the surface of the sphere, giving the impression of a huge bowl over a flat earth, covered with clouds, continents and oceans although for a real Dyson shell these would have to be immense to be noticeable. The angular size of an object at a distance d and diameter l is 2arctan(l/2d). For an object of diameter 10,000 km (like the Earth) at a distance of a 100 million km (around 120 degrees away from the observer on the shell), the angular size would be around 10^-4 rad or 0.005 degrees, roughly the size of a pea 100 meters away.
It should be noted (as Richard Treitel has pointed out) that even a very dark surface will shine intensely, making the sky much brighter than on Earth. The albedo of Earth is around 0.37, so an interior with an earthlike environment would have a sky where each patch reflects a noticeable fraction of the sunlight.
In a type I dyson sphere roughly the same things would be seen: a plane wall of orbital habitats, solar collectors and whatnot stretching away into what looks like infinity (although here the curvature may become noticeable for observant viewers) and a hemispherical bowl covering the rest of the sky, centered around the sun. Solar collectors would have a very low albedo, but it is still likely that the interior will be very bright.
7. Is a Dyson sphere stable?
In a type I Dyson Sphere all the structures orbit independently around the star, and their orbits are normal keplerian elliptic or circular orbits. Since the mass of the shell is negligible compared to the sun, the self-gravity can be ignored (it will merely cause some precession of elliptical orbits). And if two orbits intersect, they can be adjusted by using solar sails, ion engines, magsails or similar low-energy devices.
Another version would be based on statites (this is probably due to Robert L. Forward): each solar collector will also be a solar sail, and hover without orbiting above the sun, held up by light pressure. By adjusting the sail area statites can move in and out, and by adjusting their angle they can move away if needed. Traffic control may be a problem, but can likely be handled in various ways, for example by local flight control centres or automatic systems based on flocking behaviour.
The force on a statite would be F = L/(4 pi c r^2) - GMm/r^2, where L is the total luminosity of the sun (3.9e26 W), M is the mass of the sun, m is the density of the statite, r the distance to the sun and c is the speed of light. To remain in balance, the statite will have to have the density m=E/(4 pi c G M) (this assumes a 100% reflective statite). Note that this is independent of distance to the sun, closer to the sun the gravitational pull is greater, but the radiation pressure is stronger. The density depends only on the mass/luminosity of the sun. For a statite in the solar system, the density would be around 0.78 g/m^2
A rigid dyson sphere is not stable, since there is no net attraction between a spherical shell and a point mass inside. If the shell is pushed slightly, for example by a meteor hit, the shell will gradually drift off and eventually hit the star. This is a classic problem in elementary mechanics and is usually solved in introductory textbooks.
Gauss Law
One easy way to derive it is from Gauss Law: the integral of the force across an arbitrary closed surface is proportional to the amount of mass inside it. If the surface is a sphere surrounding the dyson sphere, there is obviously an inward force on the surface of the sphere since there is a mass inside it. But if the sphere is inside the dyson sphere (the sun is ignored in this calculation, as we are only interested in the gravity of the dyson sphere), there is no mass inside it and hence the integral must be zero, which means that there is no gravitational field inside the sphere.
Elementary Proof
It can also be proven using only elementary (brute force) calculus. This treatment is from Kleppner & Kolenkow, An Introduction to Mechanics (p. 101) and deals with the force between a point of mass m at radius r on the x-axis from a spherical shell centred at the origin:
Divide the shell into narrow rings. Let R be the radius of the shell, t its thickness (t << R). The ring at angle theta, which subtends an angle dtheta, has a circumference 2 pi R sin theta, width R dtheta an thickness t, which gives it a volume of dV=2 pi R^2 t sin theta d theta
and a mass of (M/2) sin theta dtheta where rho is the density of the shell.
Each part of the ring is the same distance r´ from m, and by symmetry the force from the ring is directed along the axis with no transversal component. Since the angle alpha between the force vector and the line of centres is the same for all sections of the ring, the force components along the line of centres add to give dF=G m rho dV cos alpha / r´^2
for the whole ring. This is then integrated: F = int (G m rho dV/r´2) cos alpha. By expressing cos alpha as a function of polar angle we get: F = [GMm/2] int_0^pi ( (r - R cos theta) sin theta dtheta)/(r^2 + R^2 - 2 r R cos theta)^2/3
(where int_0^pi is the integral from 0 to pi). Through the substitution u=r-Rcos(theta), du=Rsin theta dtheta we get: F = [GMm/2R] int_{r-R}^{r+R} (u du) / (R^2 - r^2 +2ru)^(3/2)
which is a standard integral resulting in: F = (GMm/2R)(1/2r^2)[sqrt(R^2-r^2+2ru)-(r^2-R^2)/sqrt(R^2- r^2+2ru)]_{r-R}^{r+R}
For r<R we get: F=(GMm/4Rr^2){(R+r)-(R-r)-(r^2-R^2)(1/(R+r)-1/(R-r))} = 0
8. How strong does a rigid Dyson shell need to be?
Very strong. According to Frank Palmer: Any sphere about a gravitating body can be analysed into two hemispheres joined at a seam. The contribution of a small section To the force on the seam is g(ravity)*d(ensity)*t(hickness)*A(rea)*cos(angle). The integral of A*cos(angle) is (pi)*R^2. So the total force is g*d*t*(pi)*R^2. Which is independent of distance, neatly enough. The area resisting the force is 2*(pi)*R*t. Thus, the pressure is g*d*R/2; this can be translated into a cylindrical tower of a given height on Earth. If that tower built of that material can stand, then the compression strain is not too great. At 1 AU, that comes to 2*([pi]*AU/YR)^2, or -- by my calculations -- in the neighborhood of 80 to 90 THOUSAND kilometers high. The tendncy to buckle, moreover, is another problem.
9. What about gravity on a rigid Dyson shell?
A nonrotating dyson shell would have just two sources of gravity: the shell itself and the star. As mentioned above, on the inside only the gravity of the star would be felt and everything would fall down into it, while on the outside there would be weak gravity (for a 1 AU sphere centred around the sun, the gravity would be 6e-3 m/s^2).
The only ways to make a rigid Dyson shell habitable on the inside would be either to provide it with some sort of antigravity (which is unlikely) or to rotate it, which would make only the equatorial band habitable unless the interior was terraced. A rotating dyson sphere would be under immense strains; see the section about the ringworld for a simple calculation. Niven pointed out that if you want to spin a Dyson sphere, it is better to build it like a film canister for reasons of structural strength, and then you have a Ringworld.
It has been suggested that one could live on the outside of the sphere, especially if the interior star is rather cool; it appears that a terrestrial environment is possible around M stars just at the end of the main sequence. Erik Max Francis gives the following derivation of this kind of sphere:
First, know the luminosity-mass relation for main sequence stars: L = k M^nu, where k is a constant of proportionality and nu is between 3.5 and 4.0. (k depends on the choice of nu, obviously.) You can find the constant k, given nu, based on the fact that the Sun has a luminosity of 3.83 x 10^26 W and a mass of 1.99 x 10^30 kg.
Second, know the gravitational acceleration: g = G M/R^2.
Third, the blackbody power law (we're approximating the star as a blackbody, which isn't too bad of an approximation): L = e sigma A T^4.
Knowing these factors, you can combine them to get an equation which relates the mass of the star to the desired temperature and gravity of the sphere: k M^(nu - 1) = 4 pi e sigma G T^4/g.
Substituting ideal conditions (g = 9.81 m/s^2, T = 300 K), you find that M must be between 0.054 and 0.079 masses solar (the variance is dependent on the variance in the exponent in the mass-luminosity relation). The end of the main sequence is at about 0.08 masses solar, for comparison.
This would produce spheres with a radius of 0.0057-0.0069 AU (852,720 - 1,032,240 km).
It might also be possible to have a biosphere between two dyson spheres (this is used in Baxter's The Time Ships).
10. Would the solar wind be a problem?
If an earthlike ecology was built inside a large rigid dyson shell, there would be an influx of ions (mostly hydrogen) from the solar wind. The solar wind has a density of around 5 ions/cm^3, moving at around 500 km/s; that would lead to an influx of 2.5e12 ions/m^2/s. This might appear large, but is actually a tiny amount, just 4e-12 mol (one gram of hydrogen is approximately one mol). Since the hydrogen could not naturally escape from the atmosphere it would gradually become more and more hydrogen rich, but it would take trillions of years before the effects became significant. The net force from the solar wind and the light pressure (which is larger than the solar wind pressure) is also minor compared to the attraction of the sun and the internal strains of a rotating dyson shell. In a type I dyson sphere the light pressure could be used to keep statites hanging in space.
It should be noted that there would be no auroras in a dyson shell, since there is no magnetic field. This also would also mean that more radiation would reach the ground from the sun since it cannot naturally be deflected (although one could imagine megaengineering systems to provide an artificial magnetic field).
11. Can a Dyson sphere be built using realistic technology?
A type I Dyson sphere can be built gradually, without any supertechnology or supermaterials, just the long-term deployment of more solar collectors and habitats. This work could start today (and one might argue that our satellites are the first step). Using self-replicating machinery the asteroid belt and minor moons could be converted into habitats in a few years, while disassembly of larger planets would take 10-1000 times longer (depending on how much energy and violence was used).
A rigid dyson shell would require superstrong materials, and its construction is complicated since half a shell is unstable. One could conceive of some dramatic capping process, where a number of previously freely orbiting structural components at the same time moved inwards to lock together into a shell (for example twenty spherical triangles). This would require tremendous precision, but since supertechnology is already assumed for building a rigid shell, it seems almost trivial. As somebody put it, if you can build a dyson shell you don't need it.
12. Is there enough matter in the solar system to build a Dyson shell?
Dyson originally calculated that there is enough matter in the solar system to create a shell at least three meters thick, but this might be an overestimate since most matter in the solar system is hydrogen and helium, which isn't usable as building materials (as far as we know today). They could presumably be fusioned into heavier elements, but if you can fusion elements on that scale, why bother with a dyson sphere?
If one assumes that all elements heavier than helium are usable (a slight exaggeration), then the inner planets are completely usable, as is the asteroid belt. Mass (1e24 kg)
Mercury: 0.33022
Venus: 4.8690
Earth: 5.8742 Moon: 0.0735
Mars: 0.64191
Asteroids: ~0.002
Sum: 11.78733e24 kg
It is a bit more uncertain how much of the outer planets is usable. Jupiter and Saturn mainly consist of hydrogen and helium, with around 0.1% of other material. Jupiter is assumed to have a rock core massing around 10-15 times the Earth, and Saturn probably contains a smaller core massing around 3 times the Earth. Uranus and Neptunus seem to be mainly rock and ice, with around 15% hydrogen, so a rough estimate would be around 50-70% usable mass. Pluto seems to be around 80% usable. Mass (1e24 kg) Usable Mass (rough estimate)
Jupiter: 1898.8 ~58
Saturn: 568.41 ~17
Uranus: 86.967 ~43
Neptune: 102.85 ~51
Pluto: 0.0129 ~0.01
Kuiper belt objects: ~0.02 ~0.016
Sum: 2657.06
Usable: ~170
(this is based on the assumption that the size distribution of the Kuiper belt mirrors the asteroid belt)
(these tables based on information from Physics and Chemistry of the Solar System by John S. Lewis and The Nine Planets by Bill Arnett)
The inner system contains enough usable material for a dyson sphere. If one assumes a 1 AU radius, there will be around 42 kg/m^2 of the sphere. This is probably far too little to build a massive type II dyson sphere, but probably enough to build a type I dyson sphere where mass is concentrated into habitats and most of the surface is solar sails and receivers, which can presumably be made quite thin.
With the extra material from the outer system, we get around 600 kg/m^2, which is enough for a quite heavy sphere (if it was all iron, it would be around 8 centimeters thick, and if it was all diamond around 20 centimeters).
A Type III shell, a "dyson bubble", would have a very low mass. Since its density is independent of the radius (see the stability section), its mass would scale as r^2. For an 1 AU bubble, the total mass needed would be around 2.17e20 kg, around the mass of Pallas.
13. Wouldn't a Dyson sphere heat up?
Even if the civilisation living in the Dyson sphere did its best to store available energy, thermodynamics eventually wins and the sphere begins to radiate away energy until equilibrium is reached. Its temperature becomes T=[E/(4 pi eta sigma r^2)]^(1/4) where eta is the emissivity (=1 for a blackbody), sigma the constant of Stefan-Bolzman's law (5.67032e-8 Wm^2K^-4)and E the total energy output of the star measured in watts.
In theory, if eta is very low the interior of the sphere could become as hot as desired, but this is unlikely since the material of the sphere would start to melt or evaporate if the temperature moved above 2000-3000K or so. And if the surface of the star became hot enough, the outer parts of the star would expand and a new thermal equilibrium set in with less internal energy production. If the sphere was a perfect energy container the star would eventually expand until its fusion processeses ended; if the temperature was lowered (by energy use) fusion would resume until an equilibrium was reached - a bottled star.
It should be noted that at 1 AU, the energy flux is around 1.4e3 W/m^2, which calculates as around 395 K, or 122 degrees C if the sphere is a blackbody. This is a bit too hot for an earthlike biosphere (Earth is cooled by its rotation, which effectively halves the energy flux, and its spherical shape, that lowers it further), and a dyson shell need some rather impressive cooling to work.
The radius of the smallest passively radiating shell with thermal tolerance T_max is r_smallest = sqr(E/(4 pi eta sigma T_max^4))
Diamond can stand around 4000K; putting 4000K into the equation, we get 1.48e9 meters, or around 1.4 million kilometers. For 1000K we get a radius of 2.37e10 meters, or around 23 million kilometers. This is roughly 2 and 32 solar radii respectively. With active cooling the shell can be made much smaller.
14. Other Dyson Sphere-Like Concepts
Ringworlds
Ringworlds were introduced by Larry Niven in Ringworld. A ringworld is essentially a band encircling a star, rotating to create gravity and covered with an ecosphere. The atmosphere is held in by gravity and 1000-kilometre mountain walls at the edges. Day and light are provided by a second ring of "shadow squares", black rectangles covering the sun during "night". Cooling systems keep the climate stable and an automated meteor defence system shoots down meteors.
Just as with a type II Dyson sphere the internal stresses would require an immensely strong material (Niven uses the invented material scrith, a greyish translucent material with strength on the order of the nuclear binding strength). The stress is F= r rho g [N/m]
where rho is the weight of the ringworld per square meter (kg/m^2) and g is the surface acceleration and r is the radius. For the ringworld g was close to earthly, a radius of around 1 AU and there was at least a kilometre of surface material of approximately eartlike density. This would provide a stress on the order of 1e18-1e19 N/m.
The ringworlds instability is also (in)famous. It is not neutrally stable like a dyson sphere, but dynamically unstable - a small disturbance (such as the inhomogenities in the solar wind or meteor strikes) will grow gradually, and the ringworld would gradually loose its centeredness until it runs into its sun (the ringworld is transversely stable, if the ring is perturbed along its axis it will oscillate around the equilibrium position). See Erik Max Francis' page abour ringworld stability for an easy derivation. Niven solves this problem in Ringworld Engineers by placing ramjets along the edges, forming an active stabilisation system.
A related idea to ringworlds is Ian Bank's orbitals. An orbital is a small ringworld orbiting the sun (instead of encircling it; this circumvents the instability), with a rotation period of 24 hours and earthlike gravity due to the spin. Its size would be r=g T^2 / (4 pi^2) which gives a radius of around 2 million kilometers. If the orbital is tilted and sufficiently broad, it could shade itself to provide day and night. Note that it still requires superstrong materials, although less extreme than scrith.
Bubbleworld, by Dani Eder < ederd@bcstec.ca.boeing.com>
A bubbleworld is an artificial construct that consists of a shell of living space around a sphere of hydrogen gas. It was invented to answer the question "what is the largest space colony that can be built". The answer is a non-rotating bubbleworld can be as large as 480,000 km in diameter (about 3 times the diameter of Jupiter), if you make certain assumptions. A rotating one is too hard for me to analyze.
Assume that you wish to have a large living volume in the form of a shell. The shell contains air, people, houses, furniture, etc. that averages 10 kg/m^3. If you fill the interior of the shell with hydrogen (the lightest gas) at room temperature, it will assume a distribution based on self-gravity. If the pressure at the inner shell boundary, where the hydrogen and living space meet, is 1 atmosphere, then there is a largest size you can build such a structure before the self-gravity of the hydrogen starts to make it smaller.
The living space has a thickness of 2400 km if you assume that the outer surface is at a pressure equal to that at 3000 m (10,000 ft) above sea-level on Earth. Such a bubbleworld would have about 5 million times the useable living volume of the Earth. The atmosphere is held in by a cap of material (such as 500 meters of iron-nickel) so as to balance the gas pressure from below. The entire structure is in pressure equilibrium, so it requires no particular structural strength.
A rotating bubbleworld would be a flattened ellipsoid and could be several times larger, but determining the shape is more complicated than the non-rotating spherical case.
The living volume of the Bubbleworld would be in a 0.001 to 0.01 gee environment, making unusual architecture and human-powered flight possible. The entire bubbleworld would mass about 3 Earths in mass.
SupraJupiter
Paul Birch suggested that Jupiter could be enclosed
in a solid shell; at a certain radius the surface gravity would be terrestial,
and energy could be provided by tapping the thermal energy of the planet.
Paul Birch "A Visit to SupraJupiter" Analog December
1992
Submerged Dyson Spheres
Nick Szabo proposed that since communications
delays are rather long in a normal-size dyson sphere and energy densities
grow as it becomes smaller, it would be advantageous to build spheres closer
and closer to the star for advanced "solid state civilizations". The logical
conclusion would be a shell around the core of the star, through which
all energy would be filtered.
The problem with this is that the amount of energy
that can be extracted from the radiation depends on the difference in temperature
on the two sides of the shell, and inside the star this will be rather
low, while outside the star the difference will essentially be between
the shell temperature and the cosmic background radiation. But it should
be noted that if neutrinous can be captured, they would provide a kind
of temperature differential that could be used (since the sun is almost
transparent to them).
Galactic Dyson Spheres
Hara Ra <harara@shamanics.com> suggested that
to maximize available energy and matter, and minimize communications delays
it would be useful for very advanced civilizations or beings ("galaxy brains")
to gather all the stars and other matter in the galaxy into a dense region
in the core.
The result would be a sphere of carefully aligned
orbiting matter just larger than its Schwartzhild radius, with a black
hole in the middle. Instead of relying on energy from stellar fusion, matter
could be fed into the black hole, releasing energy which would be used
by a surrounding "galactic dyson sphere". The total size would be on the
order of a few light-months.
Using black holes for energy production can also
be done using smaller dyson spheres. A very small black hole will radiate
intense Hawking radiation, quickly loosing its mass. If an equal amount
of mass is swallowed (for example in the form of garbage) the hole will
remain stable, and convert matter into energy which can be collected by
the dyson sphere.
15. Have any Dyson spheres been observed?
I have found the following three searches for
Dyson spheres at http://www.seti-inst.edu/searches/searches-list.html:
DATE: 1980 OBSERVER(S): WITTEBORN SITE: NASA - U OF A, MT. LEMMON INSTR. SIZE (M): 1.5 SEARCH FREQ.(MHz): 8.5 microns - 13.5 microns FREQUENCY RESOL.(Hz): 1 micron OBJECTS: 20 STARS FLUX LIMITS (W/m**2): N MAGNITUDE EXCESS < 1.7 TOTAL HOURS: 50 REFERENCE: COMMENTS: Search for IR excess due to Dyson spheres around solar type stars. Target stars were chosen because too faint for spectral type.
DATE: 1984 OBSERVER(S): SLYSH SITE: SATELLITE INSTR. SIZE (M): RADIOMETER SEARCH FREQ.(MHz): 37x10**3 FREQUENCY RESOL.(Hz): 4x10**8 OBJECTS: ALL SKY 3K BB FLUX LIMITS (W/m**2): T/T =< .01 TOTAL HOURS: 6000 REFERENCE: 27 COMMENTS: Lack of fluctuations in 3K background radiation on angular scales of 10**-2 Strd. rules out optically thick Dyson spheres radiating more than 1 solar luminosity within 100 pc.
DATE: 1987 OBSERVER(S): TARTER, KARDASHEV & SLYSH SITE: VLA INSTR. SIZE (M): 26 (9 ANTENNAS) SEARCH FREQ.(MHz): 1612.231 FREQUENCY RESOL.(Hz): 6105 OBJECTS: G357.3-1.3 FLUX LIMITS (W/m**2): TOTAL HOURS: 1 REFERENCE: COMMENTS: Remote observation (by VLA staff) of IRAS source near galactic center to determine if source could be nearby Dyson sphere. Source confirmed as OH/IR star.
In short, none have been observed yet.
References
Slysh, V. I., Search in the Infrared to Microwave for Astro- engineering Activity, in The Search for Extraterrestrial Life: Recent Developments, M. D. Papagiannis (Editor), Reidel Pub. Co., Boston, Massachusetts, 1985
Kardashev, N. S., and Zhuravlev, V. I., SETI in Russia, paper presented at the IAA/COSPAR/IAF/NASA/AIAA symposium on SETI: A New Endeavor for Humankind, The World Space Congress, Washington, D.C., August 30, 1992. To appear in a special issue of Acta Astronautica.
Jugaku, J., and Nishimura, S., A Search for Dyson Spheres Around Late-Type Stars in the IRAS Catalog, in Bioastronomy: The Search for Extraterrestrial Life, J. Heidemann and M. J. Klein (Eds.), Lectures Notes in Physics 390, Springer-Verlag, 1991
16. Web Resources
An article about dyson spheres by Sarah Voigt: Dyson Spheres: A Primer A mini-FAQ, covering the basics (at SEDS): The Ultimate Biospheres Illustration of a dyson sphere: http://www.setiquest.com/dyson.htm. Picture of F. Dyson: http://www.setiquest.com/dyson2.htm. Computer graphics of a Ringworld: http://www.rahul.net/rootbear/graphics/ringworld/index.html Images of dyson spheres: http://www.algonet.se/~aleph/Trans/Tech/Megascale/dyson_page.html The Ultimate Biospheres: http://seds.lpl.arizona.edu/nodes/NODEv4n3-10.html Transhuman Technologies, Megascale section: http://www.thehub.com.au/~mitch/extro/mega.html Megastructures in science fiction by Ross Smith: http://www.geocities.com/SiliconValley/Park/3699/sf-megastructures.html Outside Dyson spheres by Erik Max Francis: http://www.alcyone.com/max/writing/essays/outside-dyson.html
17. What has been written about Dyson Spheres?
The original papers:
Dyson, F. J., Search for Artificial Stellar Sources
of Infrared Radiation, Science, vol. 131, pp. 1667-1668, 1959
Dyson, F. J., The Search for Extraterrestrial
Technology, in Perspectives in Modern Physics (Essays in Honor of Hans
Bethe), R. E. Marshak (Editor), John Wiley & Sons, New York, 1966
Fact:
Larry Niven: "Bigger than Worlds" in A Hole In
Space (1974) and Playgrounds of the Mind. Deals with all kinds of megaengineering
structures.
Marshall T. Savage: The Millennial Project (ISBN
0-316-77163-5). Describes a plausible space-colonization scenario, involving
the construction of a type I dyson sphere.
Fiction that involves dyson spheres or linked
concepts:
(sources gathered from Usenet discussions and
"Megastructures in Science Fiction" by Ross Smith, http://www.algonet.se/~aleph/Trans/Tech/Megascale/megastruct.txt)
------------------------------------------------------------------------Star Maker (1937) by Olaf Stapledon (An enthusiastic review) The World is Round by Rothman Larry Niven: Ringworld, Ringworld Engineers and Ringworld Throne Lord Kalvan of Otherwhen by H. Beam Piper Relics episode of Star Trek: The Next Generation Cageworld 1: Search for the Sun, Cageworld 2: The Lost Worlds of Cronus, Cageworld 3: The Tyrant of Hades and Cageworld 4: Star-Search by Colin Kapp. Orbitsville (1975), Orbitsville Departure (1983) and Orbitsville Judgement (1990) by Bob Shaw Across a Billion Years by Robert Silverberg Farthest Star (1975), Wall Around a Star (1983) by Frederik Pohl & Jack Williamson The Time Ships by Stephen Baxter The Wanderer by Fritz Leiber (1967?) mentions in passing that the light from most of the stars in the inhabited galaxy are dimmed by the density of habitats orbiting them. (David Lorenzo Duffy <dlduffy@welchlink.welch.jhu.edu>)
Dyson spheres need great big walls To keep the world from spilling out They make them out of buckyballs And use gravitons for grout
Mister Skin < mrskin@mindspring.com>
Considerably more discussion of Dyson spheres is in the Dyson sphere FAQ, <URL:http://www.student.nada.kth.se/~nv91-asa/dysonFAQ.html>.
------------------------------------------------------------------------
Send corrections/additions to the FAQ Maintainer: lazio@spacenet.tn.cornell.edu
Last Update February 07 1999 @ 02:00 AM faq-admin@faqs.org
Constructing a Dyson Sphere
Some Sketches of Dyson Spheres
Early Stage in the Construction of a Dyson Sphere
![[Image of a star enclosed by several bands of solar collectors]](d_early.gif)
Solar collectors are placed in orbit around a
star, gathering energy for use in orbital colonies and factories around
them (not visible on this picture). As they become more numerous, they
are gathered into bands inclined to each other to limit shading.
Late Stage in Construction
![[Image of a the star almost completely enclosed in a shell]](d_late.gif)
The star is now almost completely enclosed by solar collectors gathering most of its output. The bands of collectors have slightly different radii, giving the system a shell-like appearance.
Some notes on these pictures
The solar collector plates and the bands are much
too big compared to their orbits and the star. In reality they would be
tiny specks, but they are exaggerated to be visible (and limit the load
on my raytracer).
The differences in radius for each belt are also
exaggerated. In reality it would be hard to distinguish the shell from
a sphere. But it looks nice!
The solar collectors will certainly not be this
reflective, at least on the inside. Most probably they will be rather dark
on the inside and reflective on the outside, covered with radiator systems.
I doubt that the orbiting collectors will be
uniform like in this image. Most probably the various parts will be built
during different eras, and reflect different uses (advanced, high-energy
input collectors in the innermost orbit, low-cost collectors gathering
scrap energy farther out).
Other Images
Drawing of a Dyson Sphere in the solar system
(Figure 1 of Detectability of Extraterrestrial Technological Activities
by Guillermo A. Lemarchand).
Ringworld Images by James W. Williams. Renditions
of the classic Niven ringworld. This is a single structure orbiting a star,
similar to a linked band in my model. It rotates so that the centifugal
acceleration gives rise 1 G at the surface. Unfortunately it requires a
hypothetical supermaterial to work...
The Chiark Home Page contains a rendition of
an orbital a la Ian Bank's Culture novels.
------------------------------------------------------------------------
Anders Main Page
Anders Sandberg / nv91-asa@nada.kth.se
Outside Dyson spheres
------------------------------------------------------------------------
Copyright © 1996 Erik Max Francis. All rights reserved.
Solid Dyson spheres have certain disadvantages. The main problem is that the gravitational attraction inside a uniform spherical shell is zero. Since solid Dyson spheres have the biosphere on the inside, this presents a problem: You need some form of gravity generators to keep the biosphere from drifting on up into the sun.
What if we put the biosphere on the outside?
Ideally we'd like Earthlike conditions on the
outside surface; that is, one
gee of gravity and a temperature of about 300
K. Can we get this with main
sequence stars?
The mass-luminosity relation [1]
L = k Mnu, (1)
where k is a constant of proportionality and nu
is a constant somewhere
between 3.5 and 4.0. (k naturally depends on
the choice of nu, clearly.)
The gravitational acceleration g for a mass M at a distance R is:
g = G M/R2. (2)
Finally, the radiation power law relates the luminosity
L to the area A and
temperature T:
L = e sigma A T4; (3)
here, e is the emissivity of the sphere, which
is a constant which varies
between 0 (for a perfect reflector) to 1 (for
a perfect absorber, also
called a blackbody).
We can then combine these three equations into
a single one relating M (the
mass of our star), T (the temperature of our
Dyson sphere), and g (the
gravitational acceleration at the surface of
our sphere). First we start by
equating L via the luminosity of the star and
the radiation power law:
L = L
k Mnu = e sigma A T4. (4)
The area A of a sphere is simply 4 pi R2, so we can write
k Mnu = 4 pi e sigma R2 T4. (5)
We can use our gravitational acceleration equation to relate R2 to M:
g = G M/R2
R2 = G M/g, (6)
and substituing that into our equation above, we find
k Mnu = 4 pi e sigma G M T4/g, (7)
and combining like terms on both sides, we reach the final equation
k Mnu - 1 = 4 pi e sigma G T4/g. (8)
Assuming our sphere appoximates a blackbody (e
~= 1) and then substituting
ideal conditions (g = 9.81 m/s2, T = 300 K),
we find that M must vary
between 0.054 and 0.079 masses solar (the variance
is caused by 3.5 <= nu <=
4.0).
By comparison, the "end of the main sequence"
-- that is, the theoretical
point at which a main sequence star is unable
to sustain itself by hydrogen
fusion -- is at about 0.08 masses solar, but
is not precisely known. Thus it
might be possible to have solid Dyson spheres
with Earthlike conditions on
their outside surfaces around the smallest hydrogen-burning
stars in the
Univrse.
One other advantage to using red dwarfs is their
immense lifespan -- the
last-massive red dwarfs can last hundreds of
billions or trillions of years.
------------------------------------------------------------------------
Footnotes.
1.
Principles of Stellar
Evolution and Nucleosynthesis, p. 40; Donald D.
Clayton; University
of Chicago Press; 1983.
------------------------------------------------------------------------
Universe
Background -- Dyson Spheres Information
-- The Orellian System
Orell: Space City --
The 'Skyhook'
Aqua: Argo --
Vulka: Smith's Landing --
Technos --
Charan: Cicely --
Harvax: Chaross --